PERIMETER AND AREA OF A CIRCLE
We know that
The area of a circle is the measurement of the region enclosed by its boundary. It is measured in square units. -ie- square centimetres or square metres etc.
Area of the circle = πr2
The perimeter of a circle is the length of its boundary. The unit measurement of perimeter is the unit of length.
Perimeter of circle = 2πr
where r is the radius of the circle.
Perimeter of a circle is known as circumference of a circle.
AREA OF SECTOR OF CIRCLE
The part of the circle inclined between two radii (OA & OB) is called sector of circle.
Area of the sector OAPB =0 /360° x πr2
& length of an arc of sector OAPB = length of arc AB =0 /360° x 2πr
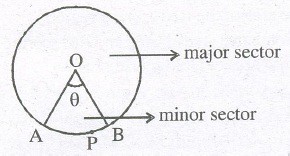
Where 0 is the measure of arc AB.
Perimeter of the sector (minor sector)
=0 /360° x 2πr + 2r
=0 /360° x 2πr + 2r
AREA OF SEGMENT OF CIRCLE
Any chord AB divides circle into two parts. The bigger part is known as major segment and smaller one is called minor segment.
Area of minor segment APB — Area of sector OAPB — area of ΔOAB
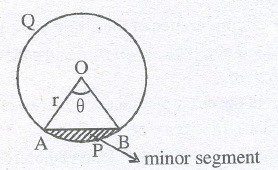
Area of major segment OAQB = πr2 — area of minor segment APB.
NOTE: Area of Δ OAB with ∠AOB = 0 = 1/2 (OA) (OB) sinθ
IN GENERAL
Area of segment of a circle = Area of the corresponding sector — Area of the corresponding triangle.
AREA ENCLOSED BY THE TWO CIRCLES
If R and r are the radii of two concentric circles such that R > r then area enclosed by the two circles = πR2 – πr2
SOME USEFUL RESULTS
(i) If two circles touch internally, then the distance between their centres is equal to the difference of their radii.
(ii) If two circles touch externally, then the distance between their centres is equal to the sum of their radii.
(iii) Distance moved by a rotating wheel in one revolution is equal to the circumference of the wheel.
(iv) The number of revolutions completed by a rotating wheel in one minute Distance moved in one minute
= Distance moved in one minute / circumference
More chapters for class 10 maths


Post a Comment