lass 12 Maths Differentiation – Get here the Notes for Class 12 Maths Differentiation. Candidates who are ambitious to qualify the Class 12 with good score can check this article for Notes. This is possible only when you have the best CBSE Class 12 Maths study material and a smart preparation plan. To assist you with that, we are here with notes. Hope these notes will helps you understand the important topics and remember the key points for exam point of view. Below we provided the Notes of Class 12 Maths for topic Differentiation.
- Class: 12th
- Subject: Maths
- Topic: Differentiation
- Resource: Notes
CBSE Notes Class 12 Maths Differentiation
Candidates who are pursuing in Class 12 are advised to revise the notes from this post. With the help of Notes, candidates can plan their Strategy for particular weaker section of the subject and study hard. So, go ahead and check the Important Notes for Class 12 Maths Differentiation
Derivative
The rate of change of a quantity y with respect to another quantity x is called the derivative or differential coefficient of y with respect to x .
Differentiation of a Function
Let f(x) is a function differentiable in an interval [a, b]. That is, at every point of the interval, the derivative of the function exists finitely and is unique. Hence, we may define a new function g: [a, b] → R, such that, ∀ x ∈ [a, b], g(x) = f'(x).
This new function is said to be differentiation (differential coefficient) of the function f(x) with respect to x and it is denoted by df(x) / d(x) or Df(x) or f'(x).
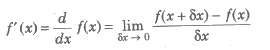
Differentiation ‘from First Principle
Let f(x) is a function finitely differentiable at every point on the real number line. Then, its derivative is given by
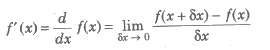
Standard Differentiations
1. d / d(x) (xn) = nxn – 1, x ∈ R, n ∈ R
2. d / d(x) (k) = 0, where k is constant.
3. d / d(x) (ex) = ex
4. d / d(x) (ax) = ax loge a > 0, a ≠ 1
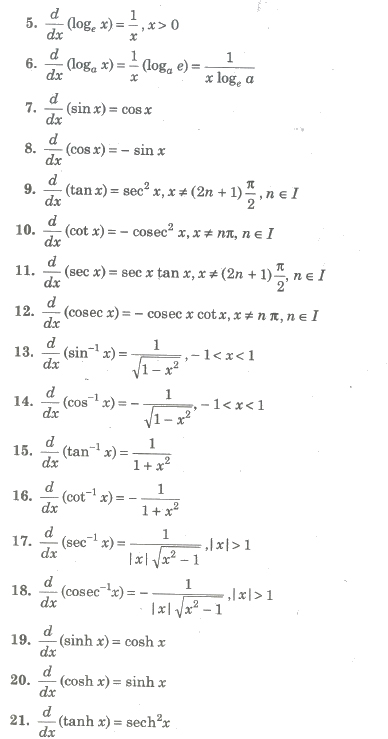
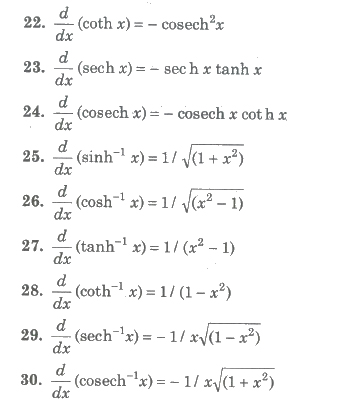
Fundamental Rules for Differentiation
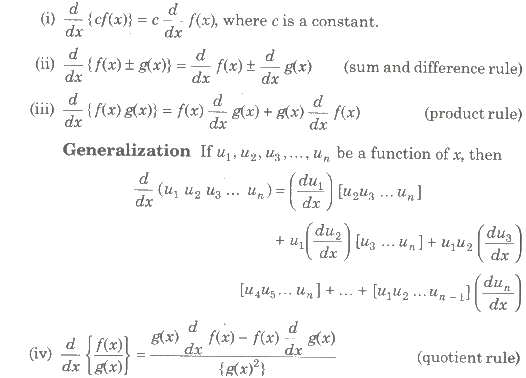
(v) if d / d(x) f(x) = φ(x), then d / d(x) f(ax + b) = a φ(ax + b)
(vi) Differentiation of a constant function is zero i.e., d / d(x) (c) = 0.
Geometrically Meaning of Derivative at a Point
Geometrically derivative of a function at a point x = c is the slope of the tangent to the curve y = f(x) at the point {c, f(c)}.
Slope of tangent at P = lim x → c f(x) – f(c) / x – c = {df(x) / d(x)} x = c or f’ (c).
Different Types of Differentiable Function
1. Differentiation of Composite Function(Chain Rule)
If f and g are differentiable functions in their domain, then fog is also differentiable and
(fog)’ (x) = f’ {g(x)} g’ (x)
More easily, if y = f(u) and u = g(x), then dy / dx = dy / du * du / dx.
If y is a function of u, u is a function of v and v is a function of x. Then,
dy / dx = dy / du * du / dv * dv / dx.
2. Differentiation Using Substitution
In order to find differential coefficients of complicated expression involving inverse trigonometric functions some substitutions are very helpful, which are listed below .

3. Differentiation of Implicit Functions
If f(x, y) = 0, differentiate with respect to x and collect the terms containing dy / dx at one side and find dy / dx.
Shortcut for Implicit Functions For Implicit function, put d /dx {f(x, y)} = – ∂f / ∂x / ∂f / ∂y, where ∂f / ∂x is a partial differential of given function with respect to x and ∂f / ∂y means Partial differential of given function with respect to y.
4. Differentiation of Parametric Functions
If x = f(t), y = g(t), where t is parameter, then
dy / dx = (dy / dt) / (dx / dt) = d / dt g(t) / d / dt f(t) = g’ (t) / f’ (t)
5. Differential Coefficient Using Inverse Trigonometrical Substitutions
Sometimes the given function can be deducted with the help of inverse Trigonometrical substitution and then to find the differential coefficient is very easy.

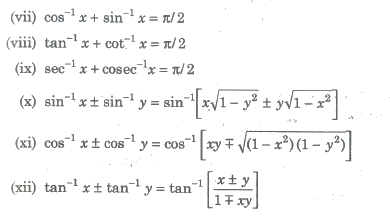
Logarithmic Differentiation Function
(i) If a function is the product and quotient of functions such as y = f1(x) f2(x) f3(x)… / g1(x) g2(x) g3(x)… , we first take algorithm and then differentiate.
(ii) If a function is in the form of exponent of a function over another function such as [f(x)]g(x) , we first take logarithm and then differentiate.
Differentiation of a Function with Respect to Another Function
Let y = f(x) and z = g(x), then the differentiation of y with respect to z is
dy / dz = dy / dx / dz / dx = f’ (x) / g’ (x)
Successive Differentiations
If the function y = f(x) be differentiated with respect to x, then the result dy / dx or f’ (x), so obtained is a function of x (may be a constant).
Hence, dy / dx can again be differentiated with respect of x.
The differential coefficient of dy / dx with respect to x is written as d /dx (dy / dx) = d2y / dx2 or f’ (x). Again, the differential coefficient of d2y / dx2 with respect to x is written as
d / dx (d2y / dx2) = d3y / dx3 or f”'(x)……
Here, dy / dx, d2y / dx2, d3y / dx3,… are respectively known as first, second, third, … order differential coefficients of y with respect to x. These alternatively denoted by f’ (x), f” (x), f”’ (x), … or y1, y2, y3…., respectively.
Note dy / dx = (dy / dθ) / (dx / dθ) but d2y / dx2 ≠ (d2y / dθ2) / (d2x / dθ2)
Leibnitz Theorem
If u and v are functions of x such that their nth derivative exist, then
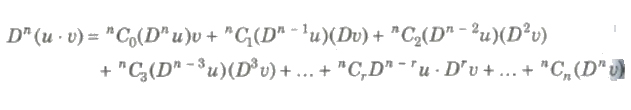
nth Derivative of Some Functions
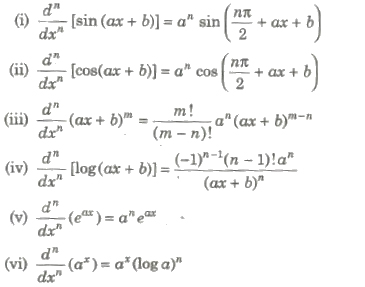

Derivatives of Special Types of Functions
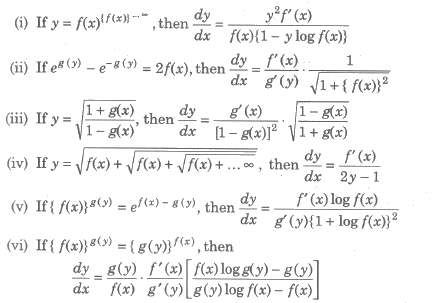
(vii) Differentiation of a Determinant
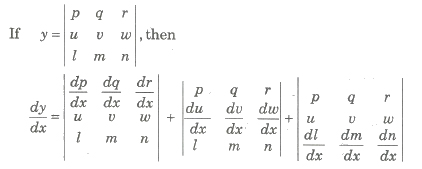
(viii) Differentiation of Integrable Functions If g1 (x) and g2 (x) are defined in [a, b], Differentiable at x ∈ [a, b] and f(t) is continuous for g1(a) ≤ f(t) ≤ g2(b), then
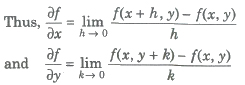
Partial Differentiation
The partial differential coefficient of f(x, y) with respect to x is the ordinary differential coefficient of f(x, y) when y is regarded as a constant. It is a written as ∂f / ∂x or Dxf or fx.
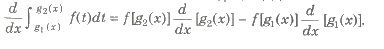
e.g., If z = f(x, y) = x4 + y4 + 3xy2 + x4y + x + 2y
Then, ∂z / ∂x or ∂f / ∂x or fx = 4x3 + 3y2 + 2xy + 1 (here, y is consider as constant)
∂z / ∂y or ∂f / ∂y or fy = 4y3 + 6xy + x2 + 2 (here, x is consider as constant)
Higher Partial Derivatives
Let f(x, y) be a function of two variables such that ∂f / ∂x , ∂f / ∂y both exist.
(i) The partial derivative of ∂f / ∂y w.r.t. ‘x’ is denoted by ∂2f / ∂x2 / or fxx.
(ii) The partial derivative of ∂f / ∂y w.r.t. ‘y’ is denoted by ∂2f / ∂y2 / or fyy.
(iii) The partial derivative of ∂f / ∂x w.r.t. ‘y’ is denoted by ∂2f / ∂y ∂x / or fxy.
(iv) The partial derivative of ∂f / ∂x w.r.t. ‘x’ is denoted by ∂2f / ∂y ∂x / or fyx.
Note ∂2f / ∂x ∂y = ∂2f / ∂y ∂x
These four are second order partial derivatives.
Euler’s Theorem on Homogeneous Function
If f(x, y) be a homogeneous function in x, y of degree n, then
x (&partf / ∂x) + y (&partf / ∂y) = nf
Deduction Form of Euler’s Theorem
If f(x, y) is a homogeneous function in x, y of degree n, then
(i) x (∂2f / ∂x2) + y (∂2f / ∂x ∂y) = (n – 1) &partf / ∂x
(ii) x (∂2f / ∂y ∂x) + y (∂2f / ∂y2) = (n – 1) &partf / ∂y
(iii) x2 (∂2f / ∂x2) + 2xy (∂2f / ∂x ∂y) + y2 (∂2f / ∂y2) = n(n – 1) f(x, y)
Important Points to be Remembered
If α is m times repeated root of the equation f(x) = 0, then f(x) can be written as
f(x) =(x – α)m g(x), where g(α) ≠ 0.
From the above equation, we can see that
f(α) = 0, f’ (α) = 0, f” (α) = 0, … , f(m – l) ,(α) = 0.
Hence, we have the following proposition
f(α) = 0, f’ (α) = 0, f” (α) = 0, … , f(m – l) ,(α) = 0.
Therefore, α is m times repeated root of the equation f(x) = 0.


Post a Comment